This is from a paper I wrote around 2004, and much of it has been incorporated into a more complete article written with Kathy Thomsen & Diane Urista in the Journal of Music Theory Pedagogy. If this sparks your interest, you should certainly check out the full article.

The solfège method of Emile Jaques-Dalcroze (1865–1950) is based entirely on the study of scales. Dalcroze believed that students who understood scales also understood musical context, and had all of the basic skills needed for dictation and sight singing. Struggling students, by contrast, got “lost” in scales or drifted into foreign keys. The Dalcroze method provides extensive experience navigating scales, so that students learn to know at all times where they are in the context of a key.
This paper discusses the sequence and methods used in teaching Dalcroze solfège. It contrasts the writings of Dalcroze on solfège pedagogy with the use of the method in current practice. Finally, it proposes applications for use in today’s aural skills and musicianship classrooms.
Emile Jaques-Dalcroze is best known as the creator of Eurhythmics, a method of music pedagogy which teaches rhythmic concepts through movement. (The influence of Eurhythmics can be seen in the Orff pedagogy, common in public schools throughout the United States.) Much has been written about Eurhythmics, and many fine primary and secondary readings are available. Today, Eurhythmics classes are taught around the world, always with the central unifying principle of connecting movement to music and vice versa.
But Eurhythmics is only the tip of the iceberg. The Dalcroze Method consists of three equally important elements: Eurhythmics, solfège, and improvisation. Together, according to Dalcroze, they comprise the musicianship training of a complete musician. In an ideal approach, elements from each subject blend together, resulting in teaching rooted in creativity and movement. Dalcroze’s solfège approach, like the rest of the method, stresses this creativity and sensitivity to musical meaning, and is especially applicable when training performing musicians.
General Background and a Note on Solfège Systems:

Dalcroze began his career as a pedagogue at the Geneva Conservatory, where he taught harmony and solfège. It was in his solfège courses that he began testing many of his influential and revolutionary pedagogical ideas. He published two major works on his solfège methods: Les Gammes et Les Tonalités, Le Phrasé et Les Nuances (Scales and Keys, Phrasing and Nuance, in three volumes) and Solfège Rhythmique (Rhythmic Solfège). Rhythmic solfège is actually a method of solfège designed to teach rhythm; it will not be discussed in this paper.
As was the tradition in Europe, Dalcroze worked in a fixed do system. In his writings on solfège, he suggests a system of fixed do that uses altered syllables to reflect sharps and flats. Sharps are given an “-è” ending, and flats an “-eu” ending.[1] Today, however, the method is taught using the more traditional form of fixed do. All types of “F,” regardless of accidental, are called “fa.” Asking students to not name the accidentals, in the Dalcroze method, has some particular advantages which will be discussed later in this paper.
Fixed do remains the least commonly used system of solfège in the United States, though many of the major conservatories prefer it. Teachers using another method of solfège, however, should not be discouraged from using Dalcroze tools. In the Dalcroze approach, the system of solfège used has much less to do with the learning than the specific exercises used. Moreover, the exercises can also be performed successfully using scale degrees, moveable do, and note names. They are particularly effective when both a fixed method (note names/fixed do) and a relational method (scale degrees/moveable do) are used.
Dalcroze’s solfège method has one main goal: developing sensitivity to function. This is done through the study of scales, scale fragments, harmonic implications of melody, and modulations. Unlike other popular ear training methods, it is not primarily a sight singing sequence. Instead, it exists to develop sensitivity to harmonic meaning. Because of this, it can be used along side of (and compliments well) many of the popular sight singing sequences.
Dalcroze Do-to-Do Scales:
Dalcroze’s major contribution to the pedagogy of solfège was the “Do-to-Do” scales. In the Dalcroze solfège method, these are the primary tool used to teach sensitivity to scale degree function. The Do-to-Do scales, sometimes known as “Dalcroze scales,” are typical scales, but sung from C (C# or Cb) up an octave, and back down:

Unlike modes, the goal of the Dalcroze scales is to hear these as scales beginning from a degree of the scale other than tonic. Though the Bb Do-to-Do scale above looks like C Dorian, a Dalcroze student would learn to hear Bb as the tonic of the scale. Singing a Dalcroze scale is somewhat like jumping into a scale that is already in progress. By learning to do this from any starting scale degree, a student becomes as familiar with every area of the scale as he or she is with the very beginning of the scale. Dalcroze minor scales are sung in the harmonic minor form. Do-to-Do scales always ends with the singing of the tonic note, generally in the octave of the scale, for closure.
Sing for yourself a C Mixolydian scale. Now sing the same notes with the harmonization below:

Now just play a V7 in F, and sing these notes again, leaping up to F at the end (for closure). Finally, sing these notes without giving yourself a starting harmony, and see if you can feel this as a scale from scale degree 5 to 5 (alternatively, hear a V7 harmony inside as you sing it).
This process — moving from a full harmonization to hearing the harmonies internally — is one that is used by many Dalcroze teachers today to introduce the Do-to-Do scales.[2] Learning to harmonize the Do to Do scales at the piano is a crucial final step in Dalcroze’s solfège method. A good harmonization directs students into singing the correct notes. Here are possible starting chords for all of the major scales:
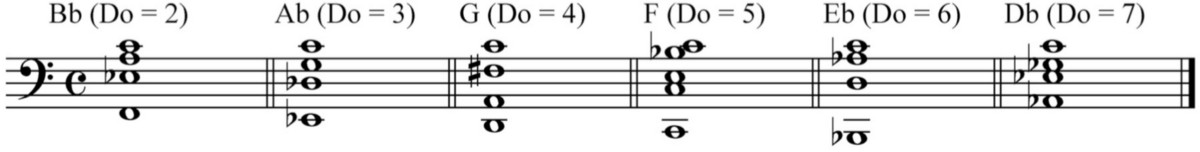
In fact, many scales may be harmonized with a pedal point and relatively few harmonic changes:

Interestingly, in his solfège texts, Dalcroze never mentions accompanying any of the scales from the piano. In fact, the steps he suggests for introducing a new Do-to-Do scale are remarkably different from how most teachers teach these today. Here are the steps Dalcroze suggests for introducing the G Major Do-to-Do scale, the first one (after C) which he introduces in Les Gammes et Les Tonalités, Le Phrasé et Les Nuances:[3]
- The teacher sings a G Do-to-Do scale, students identify how it is different from C Major. [There is an F#]
- The teacher sings the new scale, or C Major, and students identify which scale it is.
- Students sing tunes (written in the range of C to C) in C Major and then in G Major, beginning with simple patterns and moving to more complex tunes.
- Teacher sings a tune, either with or without F#. Students identify which scale it was in.
- Teacher reveals: “This is the G Major scale!” Students sing scale, followed by tonic.
- Scale is sung up and down in eighths, triplets, sixteenth notes, sextuplets (continue until tonic is reached on a beat).
- Rhythmic Patterns: Scale is sung up and down with different rhythmic patterns (quarter eighth eighth for instance).
- Listening Exercises: One student is called on to sing a melody on the board, and secretly told what scale to use. Once the other students know what scale the soloist is using, they join in singing.
- Melodies: Students sing melodies written, mostly, in the range of Do-to-Do in all of the scales they know.
It is worth noting that Dalcroze, when first introducing the scales, seems unconcerned with how students are interpreting the scales harmonically. The advantage this offers is that students must be entirely focused on the patterns of half and whole steps within the scale. Later in the sequence, Dalcroze introduces games of finding tonic, and identifying or singing specific scale degrees. Both approaches can be quite effective, and some combination of the two is probably the best option.
Advantages of Do-to-Do Scales:
The Dalcroze scales offer a number of significant pedagogical advantages. The four outlined below are, in many ways, just the most surface level advantages. Still, they present a compelling argument for incorporating Dalcroze scale work into an ear training curriculum. Compare the traditional study of scales with the study of Dalcroze scales: what do Dalcroze scales require of students that traditional scales do not?
First, students must be much more aware of exactly what accidentals are in each scale. A singer singing scales from tonic need only concern herself with where the scale begins, and then sing the same melody as she sang in C Major. When singing a Bb Major scale from Do-to-Do, by comparison, the singer must be extremely aware of the Eb and Bb. She cannot simply sing the familiar tune of the major scale. If Dalcroze scales are begun early in aural and theoretical training, they will reinforce and internalize well the study of keys and scales.
Secondly, the Dalcroze scales focus attention on the half and whole step makeup of scales. In the early stages of Dalcroze solfège study, students concentrate entirely on whether they are singing a whole step or half step. At first, singing the Ab Major Dalcroze scale requires enormous attention: a half step at the start of a scale is quite difficult for a beginner. Soon, this becomes automatic, and half steps and whole steps become aural clues for the Dalcroze student. With this knowledge, a student need only hear a tiny fragment of a scale in a composition to know immediately what part of the scale they are in. This has enormous implications for the teaching of dictation.
Thirdly, the scales teach a student to create a tonal context for themselves. In singing the scales, students learn to make C feel like any scale degree. This ability becomes quite crucial when teaching sight singing and dictation of modulating music: students must be able to make any note act as any scale degree. In the Dalcroze solfège method, this ability is hardwired from the beginning.
Finally, the scales ground in students the sound of C. Though perfect pitch should, in this author’s opinion,[4] never be a goal of ear training study, rare is the experienced Dalcroze student who cannot immediately recall the sound of a C and find any note needed by comparison to this. Moreover, the very experienced Dalcroze student in listening to a piece can immediately identify how C (and what kind of C!) is functioning within the scale of the work, and by that know what key the piece is in.
More Advanced Do-to-Do Scale Exercises:
Much of what we know of today as Dalcroze solfège pedagogy comes from other teachers who have added their own ideas to the method. The exercises and games listed in this section come both from Dalcroze’s own writings and from exercises that teachers living today have added to the method. Given his love of improvisation and innovation, Dalcroze would likely have been pleased to see how his method has grown.
Anne Farber teaches at the Dalcroze School of Music, in New York. To help her students find their bearings tonally in the scales, and to encourage them away from feeling the scales as modes, she suggests adding words and rhythms to a scale. The idea is to make the scale feel like a melody within the key, and when this is well done, it hardly feels like a piece based on a scale. One example of this might be:

The exercise is quite challenging, but is a wonderful way to master the basic forms of the Dalcroze scales. Like many Dalcroze games, it draws on another branch of the method: improvisation. In an ideal approach, the three elements (eurhythmics, solfège, and improvisation) blend together.
A number of games exist to focus students on the half and whole steps in a scale. Often this is done with rhythmic games. Students may be asked to sing a scale up and down in quarter notes, except for pairs of notes beginning with a half step, which should be sung as two eighth notes:

Or, sing a triplet for each group of notes beginning with a half step, all other notes remain quarter notes. This is tricky but fun! Try it for yourself in Bb; notice how far ahead you have to think to be able to do it. Dalcroze was particularly fond of games which demanded that sort of mental attention. Or, for a fun oddly syncopated rhythm, try singing 4 sixteenth notes for groups beginning with a half step, and quarter notes for the others.
The majority of the first volume of Dalcroze’s Les Gammes et Les Tonalités, Le Phrasé et Les Nuances is dedicated to the introduction of scales. The process he uses is essentially the same for each scale. At the end of the book, Dalcroze provides exercises which can be done in all the keys.[5] These exercises are unfortunately rarely used in the teaching of Dalcroze solfège today, and certainly deserve to be resurrected.
The exercises fall into two categories: exercises of finding or arriving at tonic, and exercises involving other scale degrees. Dalcroze specifies many variants for each of these exercises, though describing the variants is hardly necessary: they simply involve more difficult or longer patterns.
The exercises of finding tonic all begin with a scale, and lead to finding the tonic based on the scale. In the simplest form, the teacher sings a scale (up, down, or both ways) and the students sing from Do (the high or low one) up or down to tonic. In another exercise, students sing a given scale, sing up to a specified note (Sol, for instance), and then immediately sing tonic. This exercise is then repeated immediately with in a variety of keys. In another exercise, students sing a scale, and then sing a specified scale degree pattern leading to tonic (3 2 1 and 3 4 5 6 7 1 are listed as possibilities). This exercise is also to be immediately repeated in a variety of keys.
Dalcroze begins to make the bridge to dictation with one final exercise of finding tonic. Students listen to a piece in any key, the teacher plays Do, and then Dalcroze gives the advice, “Without thinking of a particular scale, simply from the influence of the tune, sing the Do-to-Do scale.” From this, the students are able to determine the key of the piece.
To this group, one might add two exercises from living Dalcroze teachers. Anne Farber plays a game in which she gives only a dominant chord with Do on top before asking students to sing a scale. They are to figure out from the chord what scale to sing. (For advanced students, they are to show with their fingers, as soon as possible, what scale degree Do occupies.) Here are possible chords (all V7’s or extended V7’s) for the major keys:

For minor keys a Vb13 can often be used (as this includes the lowered scale degree 3, which is enough information to let the student know it is a minor scale).
Lisa Parker, who directs the Dalcroze program at the Longy School of Music, has an exercise in which she improvises at the piano, keeping a C pedal present (in either an outer or inner voice). Around it she provides an accompaniment that makes clear the key. The students are to determine how C is functioning, and then they sing the Do-to-Do scale to check and see if they were right.
The exercises involving other scale degrees are largely designed to help students in finding difficult scale degree patterns. Generally, the students sing a scale up and down, followed by a given scale degree pattern which does not end on tonic. This is repeated in different keys, and with more and more difficult scale degree patterns. It is worth noting that Dalcroze’s students would have sung the scale degree patterns on fixed do, and certainly part of his goal is making sure that students know exactly how every note is functioning in every key. This is just another way in which Dalcroze uses fixed Do to teach function.
In another exercise, the students sing a scale, and then the teacher sings random notes in the scale. The students must immediately call out the scale degree number of the note sung. This is also repeated in many keys.
Finally, Dalcroze introduces his, “Marches Melodiques,” or “Melodic Walks.” Here a rhythmic or melodic pattern is given, and it is taken for a walk up or down the scale:

These patterns serve to make students very aware of the makeup of smaller parts of each scale: in fact the second and third volumes of Les Gammes et Les Tonalités, Le Phrasé et Les Nuances are largely devoted to this sort of exercise.
Together, all of these exercises using the Do-to-Do scales are designed to make students very sensitive to the different parts of the scale, and the harmonic implications of different scale degrees. Unlike systems which specifically name function (scale degrees, moveable Do), Dalcroze’s solfège method, by its use of fixed Do, intentionally obscures function. Because of this, in every Do-to-Do exercise, the main goal is to discover how Do is functioning. Although it is a “fixed Do” method, Dalcroze’s solfège method exists primarily to teach functional hearing.
Students who study these Do-to-Do scales learn to hear function within different keys. The process of converting from note names to scale degrees becomes automatic, so that a Dalcroze student hearing Do in Bb knows immediately how it feels in the key. Moreover, the experienced Dalcroze student knows how every pitch feels in every key, and knows this in terms of the note names without having to think about the scale degrees they occupy. This allows students to transfer that knowledge into performance, as they know just how any note they will play will feel, and adjust their playing accordingly.
Exercises of Scale Fragments:
The study of the Do-to-Do scales makes up the first component of Dalcroze’s solfège method. It is the centerpiece of the method. Once students have begun to grasp the Dalcroze scales as a whole, they then begin to explore within the scales. The second and third volumes of Les Gammes et Les Tonalités, Le Phrasé et Les Nuances are devoted entirely to the study of scale fragments.
The exercises begin with fragments of two notes and continue all the way up to fragments of seven notes. This is the sole connection to “interval” in the method. Students learn to hear intervals as omitted steps within scales. A third is simply a skipped step. In Dalcroze terms, these fragments are known as dichords, trichords, tetrachords, and so on. These are, of course, not to be confused with the meaning of these terms in set theory. A tetrachord, in Dalcroze terms, is any four adjacent notes of a scale.
Exercises of scale fragments fall into several categories: singing, identification, and improvisation. The goal is to immediately recognize and be able to sing fragments, and to know where in the scale these fragments exist. A simple exercise using dichords may make this more clear. Students are asked to sing a dichord pattern up the scale (Do Re, Re Mi, etc…) in eighth notes. When they come to a half step dichord, they are to sing a triplet figure (Mi Fa Mi). For instance, in E Major:

For added fun students can pat their laps on the beat and clap on the off beat eighth notes, so they will end up singing several 3 against 2 patterns. (The E Major Dalcroze scale, of course, looks like a C# natural minor scale. However, after enough work with the Do-to-Do scales has been done, a Dalcroze student is capable of hearing a harmonization internally that makes this feel like E Major. The teacher can help this along by harmonizing it in E as the students perform the exercise.) Doing this dichord exercise in all of the Do-to-Do scales hardwires the locations of the half steps in the scale, and helps build even further functional awareness.
Most Dalcroze teachers spend more time on trichords than any other scale fragment. Identification games and improvisation games are particularly popular with the trichords. To ease in identification games, Bob Abramson has given numbers to the trichords that occur:

The first three occur in both major and minor, the last two occur only in harmonic minor. In Abamson’s solfège classes, students often answer by showing the trichord number with their fingers. In a common exercise, students show the trichord with their fingers while singing up a scale in trichords:

Sing the above example while showing trichords, and then pick any other Dalcroze scale, and do the same exercise. You will notice how very aware you become of the order of half and whole steps. Alternatively, instead of singing solfège sing, “Trichord 1, trichord 2,” and so on. Trichords may also be sung out of order (Mi Do Re, Fa Re Mi, etc…), for an additional challenge. Students might also be asked to sing a different rhythmic pattern for each trichord, perhaps:

This particular rhythmic pattern reinforces the location of the half steps with a longer note value on the way up. You could reverse these on the way down, or enjoy the mental disjunction when the quarter notes no longer line up with the half steps. (Dalcroze particularly enjoyed this sort of cognitive dissonance, and in Eurhythmics he has an entire set of games designed to teach the skill of “dissociation.”)
Outside of the context of scales, students might be asked to sing particular trichords from any given note. This exercise is useful when learning to sing the Do-to-Do scales, as singing the first trichord is often the most difficult step.
Beyond these exercises of singing trichords, there are many exercises of identification. In the simplest version, which carries down straight from Dalcroze, a teacher sings a scale fragment and a student identifies the makeup of the fragment and what scale degrees it could occupy in major or minor.
Lisa Parker teaches an identification exercise that is a combination of trichord and Do-to-Do work. She improvises a tune/piece that cadences on C. Students (in groups of three) arrange themselves in space to reflect the opening trichord of the Dalcroze scale (for Ab, for instance, two students might stand close, with the third farther away). The students then sing the Do-to-Do scale. This is an especially fun game, as it requires teamwork, movement, and extreme concentration.
Anne Farber sometimes will have pairs of students improvise together in different trichords of a scale. For instance, one student might be given 5, 6 and 7 in (harmonic) minor, while another might be given 7, 1 and 2. Together the students create a little duet, staying within their trichords.
Similar exercises can be done with tetrachord, pentachords, hexachords, and heptachords, though few Dalcroze teachers today work with the larger scale fragments. It is not clear that there is much need for them if trichords are understood well.
Exercises in Harmony:
Dalcroze himself does not discuss harmonic aural training in his writings on solfège. However, many Dalcroze teachers today do teach harmonic hearing using Dalcroze-like methods.
Many teachers use movement to represent different harmonies. Daniel Cataneo, who works with Robert Abramson at the Robert Abramson Dalcroze Academy, has students sit solidly in a chair during tonic harmonies. For dominant harmonies, the students lean forward, longingly. On pre-dominant harmonies, they lean slightly to the side. Lisa Parker plays a similar game: she improvises a waltz, first in tonic and dominant, and students find a different type of waltz step for each. Then she introduces subdominant, and the students find a third step for it. The game is then to waltz in real time to her improvisation, adjusting the waltz step to fit the harmony.
There is a long tradition of transferring Bach Chorales into movement in Dalcroze circles. In one such exercise, Lisa Parker has students arrange themselves (in groups of four) in space — as if they were on a giant grand staff. Students learn a chorale well, and then move it together, so as to notice how the lines pull against one another.
A third approach to harmony, based on improvisation, comes from Jean-Marc Aeschimann, who teaches at the Dalcroze Institute in Geneva. He will write out a harmonic progression on the board in tight spacings, and have students arpeggiate up and down each harmony. Students then perform the progression as a random chorale: each picking his or her own smooth path through the harmonies. Then, students come up with a pattern to use within each harmony (bottom, top, middle, top — for instance) and add a rhythm to this pattern. Several complimentary patterns are put together, and finally, students are asked to take a melodic solo over the harmonies. When done well, one harmonic progression can last nearly an entire hour. By the end of the time, students hear very well the harmonies internally.
Exercises in Modulation:
Dalcroze hints at modulation in his introduction to Les Gammes et Les Tonalités, Le Phrasé et Les Nuances but unfortunately never gets around to presenting exercises. Dalcroze viewed modulation as a change of scale, and felt that if students could immediately swap between any given scale and another scale, they could handle all modulations. The majority of the exercises that follow are of my own invention, though they are very clearly in the tradition of the Dalcroze scales, and only slightly extend the existing work.
It is possible to harmonize a Dalcroze scale in such a way that it convincingly modulates from one key to another. A class might be instructed to sing a Do-to-Do scale, be given a starting C7 chord, and then have this curve ball given them:
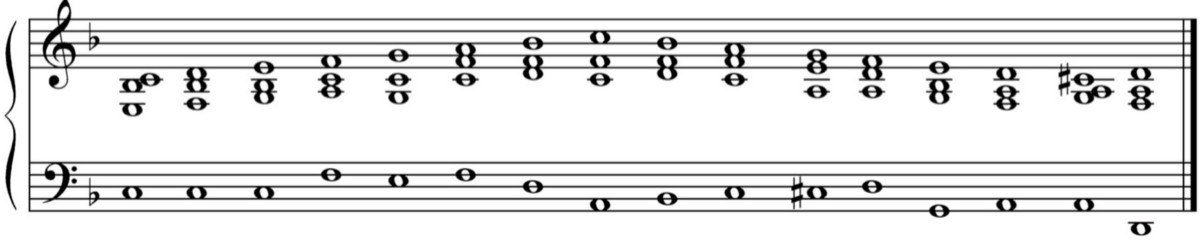
The harmonization is clear enough that, nine out of the ten times I have tried this, the class has automatically sung the C# at the bottom of the scale. The trick is then isolating on which note they feel it shift into a new key. Some students will argue that they feel it at G, but most feel it at the E. I have the class sing the scale again, and this time I give them only the starting C7 harmony, the pre-dominant and first chord of the cadential 6/4 from the piano. Finally I have the students sing the scale without any harmonization, and encouraging them to remember the harmonic support at the pivot. These sorts of harmonizations are possible for all of the typical modulations (to and from V in Major, and to and from III in minor), though they take a bit of thought to work out.
In another exercise, students sing up a Dalcroze scale to a given note. They put a fermata on that note, at which point a new scale degree is called out. The students complete the Do-to-Do scale and sing the new tonic. For a more challenging version, after the fermata, the students complete the Do-to-Do scale silently in their head and all sing the tonic together. Or, for even more fun, the students sing the new tonic immediately after the fermata.
As a middle step leading to this, I often have students first learn to think of any tone as being 5 in a new key. Students sing a Dalcroze scale to a given point, and then sing “5 4 3 2 1.” At that moment, I at first give them the harmonic support of a dominant pedal, and then encourage them to hear this harmony for themselves. To test whether they are hearing it, I often have them arpeggiate it (“5 4 2 7 5”) before going on. Once students have solidly mastered this, I like to use the “Christmas Song” by Mel Torme, as it features several examples of just this sort of pivot.[6]
First, try singing the first two A phrases of the song form in fixed Do solfège in C Major. If you’re not very careful, you’ll run into a trap, and you will wind up calling the first note of the second phrase “B,” though it’s the exact same note you started on! To solve this, I have students hold fermatas on the pivot tones and ask them to tell me what scale degree the note feels like (in this tune, it becomes 5 each time). We then decide how we would spell them, and sing the tune again on solfège:

Students who have gone through the Do-to-Do training will be able to feel that each of these notes becomes five, even without the harmonic support of the piano underneath.
A final game of harmonization comes from Herb Henke, emeritus professor of music education and Eurhythmics at Oberlin. Take the song “My Country ’tis of Thee,” begin each new phrase on the last note of the previous phrase. Sing the entire song this way (you’ll want to start in a fairly high key). Then repeat the process, at each phrase, show with your fingers what scale degree that note will become in the new key. Finally, at each phrase, hold the last note until you can make it feel like the new scale degree in your mind before going on.
In all of these games, the mind must provide a new harmonic context for the note which is sung before going on. This is, in many ways, the final goal of Dalcroze’s solfège method: the ability to instantly make any note feel like any scale degree, and the ability to recognize immediately what function any note has within a scale.
Dalcroze Solfège Today:
Throughout this paper there have been few references to sight singing or dictation. Dalcroze certainly intended these to be elements of a solfège class: he discusses methods for giving a melodic dictation, in fact.[7] In addition, Dalcroze published separate collections of tunes for solfège classes. But he gives little advice for teaching these skills. This is perhaps the greatest strength of Dalcroze’s solfège method. While numerous methods exist for teaching sight singing (most well known, perhaps, is the Kodaly method), no other method exists solely to teach such sensitivity to the function and harmonic meaning of tones.
The Dalcroze exercises can easily be incorporated into an existing curriculum, and work well as a “spice.” This author has found that, particularly in the first semester of aural skills study, sprinkling in work on the Do-to-Do scales has an enormous positive impact, especially for students who struggle with intonation and tend to drift into foreign keys. The method makes it almost impossible for a student to accidentally sing a note outside of the scale they are working in.
Dalcroze’s method requires no books and no paper. As a result, it is available immediately to any teacher willing to experiment! So, go ahead, try some of the exercises in this paper for yourself. Accustom yourself to the kind of thinking they require, and then try them out with your students. The rewards, in terms of improved functional hearing, are well worth it!
Notes:
[1] Jaques-Dalcroze, Emil. Les Gammes et Les Tonalités, Le Phrasé et Les Nuances. Lausanne, Jobin. c1923., p. 14
[2] I learned this sequence primarily from Lisa Parker and Anne Farber in studies at the Longy School. Dalcroze never specifically discusses accompanying solfège lessons at the piano, but nearly all Dalcroze teachers today do so. It is unclear whether Dalcroze himself would have accompanied these solfège exercises.
[3] Dalcroze, pp. 27–29.
[4] Dalcroze does not specifically discuss absolute pitch. However, in his introduction to Les Gammes…, he insists that a solfège student should be able to recall any pitch at any moment by reference to C natural. This seems to me a blending of absolute and relative hearing, with the emphasis on relative hearing.
[5] Dalcroze, pp. 90–107.
[6] The solfège/spelling issues of this particular song were first pointed out to me by Bill Marvin of the Eastman School of Music.
[7] Dalcroze, p. 9

